-

-
Courses
Find courses by:
Collections
Cross-Disciplinary Topic Lists
- About
- Donate
- Featured Sites
This is an archived course. A more recent version may be available at ocw.mit.edu.
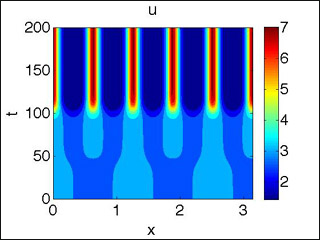
Turing pattern in a 1D reaction-diffusion system. (Image by Dr. Aslan Kasimov.)
Dr. Aslan Kasimov
18.311
Spring 2009
Undergraduate
This course is about mathematical analysis of continuum models of various natural phenomena. Such models are generally described by partial differential equations (PDE) and for this reason much of the course is devoted to the analysis of PDE. Examples of applications come from physics, chemistry, biology, complex systems: traffic flows, shock waves, hydraulic jumps, bio-fluid flows, chemical reactions, diffusion, heat transfer, population dynamics, and pattern formation.
Aslan Kasimov. 18.311 Principles of Applied Mathematics, Spring 2009. (Massachusetts Institute of Technology: MIT OpenCourseWare), https://ocw.mit.edu (Accessed). License: Creative Commons BY-NC-SA
For more information about using these materials and the Creative Commons license, see our Terms of Use.
